Ohm's Law Calculator
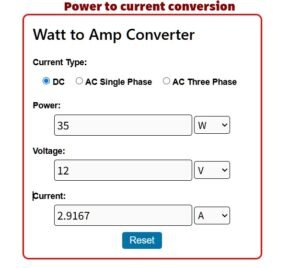
Also Try–Watt to ampere converter
What Exactly is Ohm’s Law?
Imagine electricity flowing through a wire like water through a pipe.
- Voltage (V) is like the pressure pushing the water. It’s the electrical potential difference that drives the flow of charge. Voltage is measured in Volts (V).
- Current (I) is the rate of flow of water. It’s the amount of electrical charge flowing past a point per unit of time. Current is measured in Amperes (A), often shortened to Amps.
- Resistance (R) is like the narrowness or roughness of the pipe, hindering the flow of water. It’s the opposition to the flow of electric current. Resistance is measured in Ohms (Ω) (the Greek letter Omega).
Ohm’s Law states that the current flowing through a conductor between two points is directly proportional to the voltage across the two points and inversely proportional to the resistance of the conductor.
Simply put:
- More voltage means more current (if resistance stays the same).
- More resistance means less current (if voltage stays the same).
This fundamental relationship is the essence of Ohm’s Law.
The Power of the Formula: V = IR
The relationship described by Ohm’s Law can be elegantly expressed in a simple yet powerful formula:
V = I × R
Where:
- V represents Voltage (in Volts)
- I represents Current (in Amperes)
- R represents Resistance (in Ohms)
This formula allows us to calculate any one of these three quantities if we know the other two.
The Ohm’s Law Triangle: A Handy Tool
To easily remember and rearrange the formula, many people use the Ohm’s Law triangle:
V
/ \
/ \
I --- R
To find any of the variables, simply cover it with your finger:
- To find Voltage (V): Cover V, and you’re left with I × R. So, V = I × R.
- To find Current (I): Cover I, and you’re left with V above R. So, I = V / R.
- To find Resistance (R): Cover R, and you’re left with V above I. So, R = V / I.
This triangle is a fantastic visual aid for quickly applying Ohm’s Law in various scenarios.
Ohm’s Law in Action: Real-World Examples
Let’s explore some practical examples to solidify your understanding of Ohm’s Law:
Example 1: The Simple Light Bulb
Imagine a light bulb connected to a 12-volt battery. If the bulb has a resistance of 4 Ohms, how much current will flow through it?
Using the formula I = V / R:
I = 12 V / 4 Ω = 3 Amperes
Therefore, a current of 3 Amperes will flow through the light bulb.
Example 2: Adjusting the Brightness
Suppose you have a circuit with a constant voltage of 9 Volts. If you want to increase the brightness of a light bulb (which means more current), what should you do to the resistance?
According to Ohm’s Law (I = V / R), to increase the current (I) while keeping the voltage (V) constant, you need to decrease the resistance (R). This is why dimmer switches work by changing the resistance in the circuit.
Example 3: Protecting a Circuit with a Fuse
A device designed to operate at 5 Volts draws a maximum current of 1 Ampere. What is the minimum resistance it should have to prevent damage?
Using the formula R = V / I:
R = 5 V / 1 A = 5 Ohms
The device should have a minimum resistance of 5 Ohms. This is crucial for selecting the appropriate fuse for circuit protection. If the resistance drops too low, the current will exceed 1 Ampere, and the fuse (a component designed to break the circuit at a certain current) will blow, protecting the device.
Example 4: Understanding Voltage Drop
In a long extension cord with a resistance of 0.5 Ohms, a current of 2 Amperes is flowing. What is the voltage drop across the extension cord?
Using the formula V = I × R:
V = 2 A × 0.5 Ω = 1 Volt
This means that there is a 1-Volt drop in electrical potential across the extension cord due to its resistance. The voltage available at the end of the cord will be 1 Volt less than the voltage at the source.
Ohm’s Law Limitations
While Ohm’s Law is incredibly useful, it’s important to remember that it applies primarily to ohmic materials. These are materials where the resistance remains relatively constant over a range of voltages and currents (like many metals at a constant temperature).
Some materials and devices, like diodes and transistors, do not exhibit this linear relationship and are considered non-ohmic. In these cases, Ohm’s Law cannot be directly applied.
Furthermore, factors like temperature can affect the resistance of a material. For example, the resistance of a filament in an incandescent light bulb increases as it heats up.
Why is Ohm’s Law So Important?
Ohm’s Law is a foundational concept in the study of electricity and electronics. It has countless applications, including:
- Circuit Design: Engineers rely on Ohm’s Law to design circuits with the correct voltage, current, and resistance values for components to function properly and safely.
- Troubleshooting: When electrical devices malfunction, understanding Ohm’s Law helps technicians diagnose problems by measuring voltage, current, and resistance at different points in the circuit.
- Power Calculations: Ohm’s Law is closely related to the concept of electrical power (P = V × I). By understanding the relationship between voltage and current, we can determine the power consumed or delivered by a circuit.
- Everyday Electronics: From understanding why a battery powers a device to figuring out the correct resistor for an LED, Ohm’s Law is at play in the background of countless electronic devices we use daily.
Conclusion:
Ohm’s Law, with its simple formula and profound implications, is a cornerstone of understanding electricity. By grasping the relationship between voltage, current, and resistance, you’ve taken a significant step in demystifying the world of electronics. Remember the formula (V = IR) and the handy Ohm’s Law triangle, and you’ll be well-equipped to analyze and understand basic electrical circuits. Keep exploring, and you’ll discover even more fascinating aspects of this essential force that powers our lives!
More tools
- capacitor code to value and value to code converter
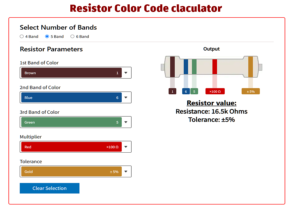
- Resistor color code calculator
- Battery charging time calculator
- capacitor unit converter